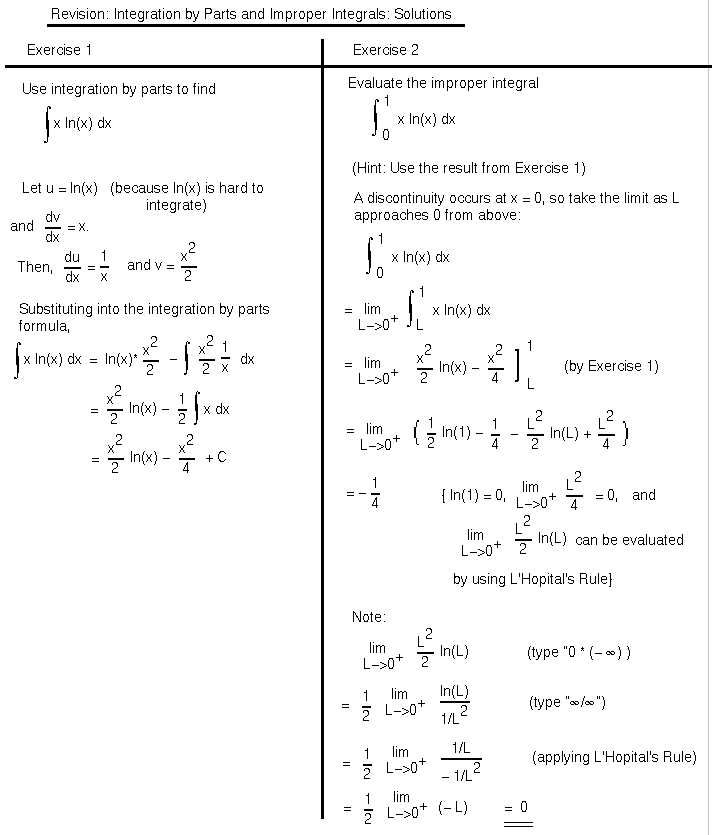
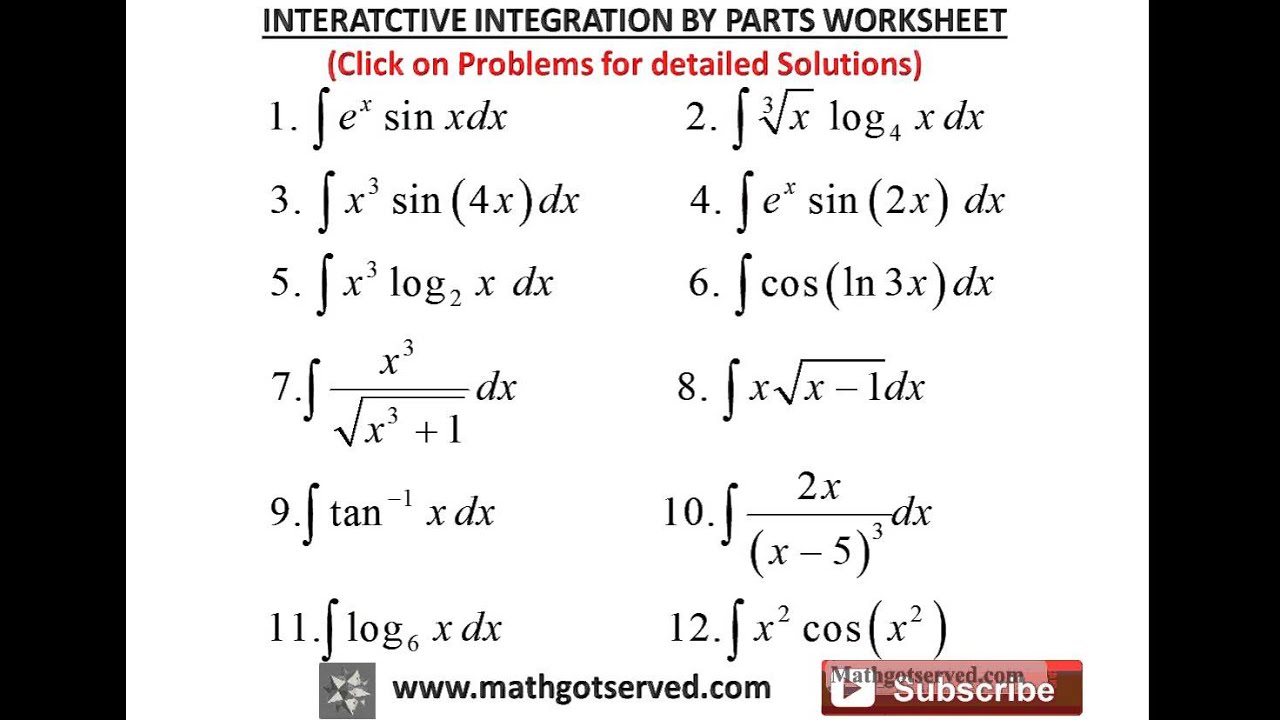
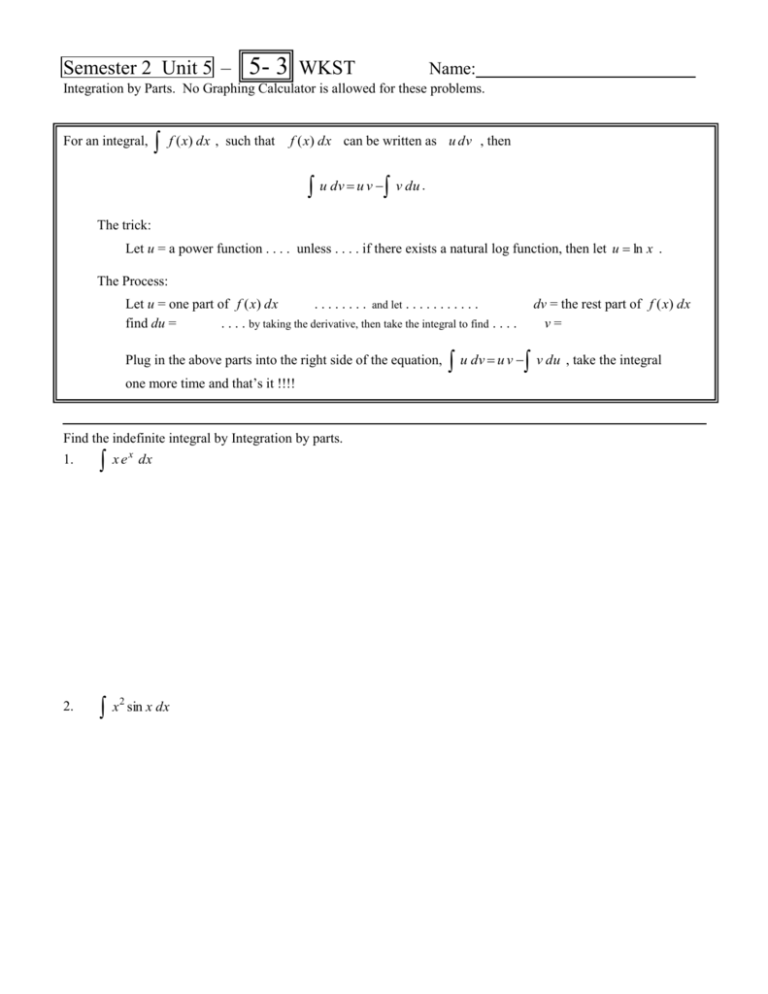
Integration By Parts Worksheet

Integration By Parts Worksheet - ∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1. (x + 1/x)² = x² + 2 + 1/x². Web integration by parts is the technique to integrate the functions when typical integration does not work. (1) u= ex dv= sin(x) du= exdx v= −cos(x) (2) u= ex dv= cos(x) du= exdx v= sin(x) exsin(x)dx= −excos(x)+. (1) integrate the following with respect to x: You can actually do this problem without using integration by parts. We choose dv dx = 1 and u = ln|x| so that v = z 1dx = x. Web in using the technique of integration by parts, you must carefully choose which expression is u. Web solve the following integrals using integration by parts. Write an expression for the area under this curve between a and b.

$Integration By Parts Exercises With Answers Pdf Online degrees$

Integration By Parts Exercises With Answers Pdf Online degrees

This technique is useful when one function can be differentiated repeatedly, and other function can be integrated repeatedly. Use the substitution w= 1 + x2. ∫ f ( x) g ( x) d x. Web we can use the formula for integration by parts to ﬁnd this integral if we note that we can write ln|x| as 1·ln|x|, a product..

Integration By Parts Worksheet —

Web integration by parts worksheet. Then dw= 2xdxand x2 = w 1: Use the substitution w= 1 + x2. Now that we have used integration by parts successfully to evaluate indefinite integrals, we turn our attention to definite integrals. Web section 7.1 :

integration by parts interactive worksheet YouTube

(1) u= ex dv= sin(x) du= exdx v= −cos(x) (2) u= ex dv= cos(x) du= exdx v= sin(x) exsin(x)dx= −excos(x)+. Write an equation for the line tangent to the graph of f at (a,f(a)). I did it correctly, but i was just wondering is there a way to do a kind of u. This technique is useful when one function.

$Integration By Parts Exercises With Answers Pdf Exercise$

Integration By Parts Exercises With Answers Pdf Exercise

∫ f ( x) g ( x) d x. Web for the definite integration by parts worksheet, i was doing one that was: Use the substitution w= 1 + x2. (1) integrate the following with respect to x: Web solve the following integrals using integration by parts.

Pin on Worksheet Sample

∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1. I did it correctly, but i was just wondering is there a way to do a kind of u. Now you can integrate each term individually:. Web integration by parts worksheet. We choose dv dx = 1 and u = ln|x| so that v.

Integration By Parts Worksheets With Answers Worksheets Master

We choose dv dx = 1 and u = ln|x| so that v = z 1dx = x. Web section 7.1 : ( 2 − 3 x) d x solution. You can actually do this problem without using integration by parts. ∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1.

Integration by Parts I Worksheet

(2) integrate the following with respect to x: Web integration by parts is the technique to integrate the functions when typical integration does not work. Web integration by parts for definite integrals. ∫ f ( x) g ( x) d x. ( 2 − 3 x) d x solution.

$Calculus Worksheets Indefinite Integration for Calculus Worksheets$

Calculus Worksheets Indefinite Integration for Calculus Worksheets

Web for the definite integration by parts worksheet, i was doing one that was: Web section 7.1 : Ap®︎/college calculus bc > unit 6. (2) integrate the following with respect to x: ∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1.

Calculus Worksheets Indefinite Integration for Calculus Worksheets

Evaluate each of the following integrals. Use the substitution w= 1 + x2. Web we can use the formula for integration by parts to ﬁnd this integral if we note that we can write ln|x| as 1·ln|x|, a product. For this method, the integrand is of the form. I did it correctly, but i was just wondering is there a.

Quiz & Worksheet How to Use Integration By Parts

∫ f ( x) g ( x) d x. This quiz/worksheet combo will test your ability to use integration by parts to solve problems. Web integration by parts is the technique to integrate the functions when typical integration does not work. Web integration by parts worksheet. (1) integrate the following with respect to x:

For this method, the integrand is of the form. Web section 7.1 : Web it's always simpler to integrate expanded polynomials, so the first step is to expand your squared binomial: Then dw= 2xdxand x2 = w 1: You can actually do this problem without using integration by parts. Z x3 p 1 + x2dx= z xx2 p 1 + x2dx= 1. Given r b f(g(x))g0(x) dx,. Web in using the technique of integration by parts, you must carefully choose which expression is u. Web solve the following integrals using integration by parts. ( 2 − 3 x) d x solution. ∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1. (x + 1/x)² = x² + 2 + 1/x². Write an expression for the area under this curve between a and b. This technique is useful when one function can be differentiated repeatedly, and other function can be integrated repeatedly. ∫ f ( x) g ( x) d x. Web we can use the formula for integration by parts to ﬁnd this integral if we note that we can write ln|x| as 1·ln|x|, a product. Web for the definite integration by parts worksheet, i was doing one that was: For each of the following problems, use the guidelines in this. Use the substitution w= 1 + x2. ∫ 4xcos(2 −3x)dx ∫ 4 x cos.

Web In Using The Technique Of Integration By Parts, You Must Carefully Choose Which Expression Is U.

I did it correctly, but i was just wondering is there a way to do a kind of u. Web we can use the formula for integration by parts to ﬁnd this integral if we note that we can write ln|x| as 1·ln|x|, a product. You can actually do this problem without using integration by parts. These worksheets precede gradually from.

Write An Equation For The Line Tangent To The Graph Of F At (A,F(A)).

Integration by parts questions 1. Now that we have used integration by parts successfully to evaluate indefinite integrals, we turn our attention to definite integrals. Web integration by parts is the technique to integrate the functions when typical integration does not work. Then dw= 2xdxand x2 = w 1:

Web We Need To Apply Integration By Partstwicebeforeweseesomething:

Use the substitution w= 1 + x2. This quiz/worksheet combo will test your ability to use integration by parts to solve problems. For this method, the integrand is of the form. (1) integrate the following with respect to x:

∫ F ( X) G ( X) D X.

Now you can integrate each term individually:. (2) integrate the following with respect to x: We choose dv dx = 1 and u = ln|x| so that v = z 1dx = x. Web for the definite integration by parts worksheet, i was doing one that was: