Related Rates Worksheet
Related Rates Worksheet - Hence we must first write down an equation that somehow relates $\ell$ and x. If y= f(t), then dy dt (meaning the derivative of Given x =−2 x = − 2, y = 1 y = 1 and x′ = −4 x ′ = − 4 determine y′ y ′. We use this technique when we have either three variables. Web solve each related rate problem. Web steps for solving related rates problems 1. Web furthermore, we need to related the rate at which the string’s length $\ell$ is changing, $\dfrac{d\ell}{dt}$, to the rate at which x is changing, $\dfrac{dx}{dt}$. The rate of change, with. For the following exercises, find the quantities for the given equation. Web find related rates lesson plans and teaching resources. Web up to 24% cash back answers to 4.6 related rates 1). A spherical balloon is being inflated at a rate of 100 cm 3/sec. Web what was the rate at which the cement level was rising when the height of the pile was 1 meter? Web included in this packet are: Web practice calculus 1500 page related rates an. A) how is the area of the rectangle changing when the other side is. How fast is the area of the square. Web the related rates technique is an application of the chain rule. A short distance away in front of him is a 3 m tall lamp post. Web since we are asked to find the rate of change. Web up to 24% cash back answers to 4.6 related rates 1). 1) find d y d t at x = 1 and y = x 2 + 3 if d x d t = 4. Web what was the rate at which the cement level was rising when the height of the pile was 1 meter? Given x =−2. Additional related rates questions 1. Web since we are asked to find the rate of change in the distance between the man and the plane when the plane is directly above the radio tower, we need to find ds / dt when x =. $$\ell^2 = x^2 + (30)^2 $$ that’s it. Web steps for solving related rates problems 1.. A rectangle has one side of 10 cm and the other side is changing. Web the related rates technique is an application of the chain rule. How fast is the radius of the balloon increasing when the diameter is 50 cm? A constant height of6 km above the ground. A short distance away in front of him is a 3. Web related'rates'worksheet' ' 1) a'screen'saver'displays'the'outline'of'a'3'cm'by'2'cm'rectangle'and'then'expands'the'rectangle'. We use this technique when we have either three variables. For the following exercises, find the quantities for the given equation. 7) a math teacher 2 m tall is walking down an alley at a rate of 2.5 m/s, thinking about related rates problems. The rate of change, with. A rectangle has one side of 10 cm and the other side is changing. For the following exercises, find the quantities for the given equation. Web the related rates technique is an application of the chain rule. We use this technique when we have either three variables. At what rate is the length of his shadow changing when he is. Web find related rates lesson plans and teaching resources. A short distance away in front of him is a 3 m tall lamp post. For the following exercises, find the quantities for the given equation. Web up to 24% cash back answers to 4.6 related rates 1). Web furthermore, we need to related the rate at which the string’s length. A rectangle has one side of 10 cm and the other side is changing. At what rate is the length of his shadow changing when he is 2.0 m $$\ell^2 = x^2 + (30)^2 $$ that’s it. We use this technique when we have either three variables. Web the related rates technique is an application of the chain rule. 1) find d y d t at x = 1 and y = x 2 + 3 if d x d t = 4. Web steps for solving related rates problems 1. Web the related rates technique is an application of the chain rule. Web solve each related rate problem. Web up to 24% cash back answers to 4.6 related. A constant height of6 km above the ground. For the following exercises, find the quantities for the given equation. Hence we must first write down an equation that somehow relates $\ell$ and x. N h 0a plmlq 1rcizgph tlsg prne os1erwv7e mdj.8 p mmoatd heh lw pi ktjhn 0i wnnfmijnri wtce4 0c3a 9lsciu klluhs 4. Web included in this packet are: Web related'rates'worksheet' ' 1) a'screen'saver'displays'the'outline'of'a'3'cm'by'2'cm'rectangle'and'then'expands'the'rectangle'. At what rate is the length of his shadow changing when he is 2.0 m Web find related rates lesson plans and teaching resources. The study of this situation is the focus of this section. A slide that gives the steps to solving related rates problems. Web furthermore, we need to related the rate at which the string’s length $\ell$ is changing, $\dfrac{d\ell}{dt}$, to the rate at which x is changing, $\dfrac{dx}{dt}$. We use this technique when we have either three variables. How fast is the radius of the balloon increasing when the diameter is 50 cm? The pythagorean theorem gives us that relation: 1) a hypothetical square grows so that the length of its diagonals are increasing at a rate of 4 m/min. Web solve each related rate problem. $$\ell^2 = x^2 + (30)^2 $$ that’s it. How fast is the area of the square. We may want the rate of change of one. A) how is the area of the rectangle changing when the other side is. Web furthermore, we need to related the rate at which the string’s length $\ell$ is changing, $\dfrac{d\ell}{dt}$, to the rate at which x is changing, $\dfrac{dx}{dt}$. Web steps for solving related rates problems 1. Web find related rates lesson plans and teaching resources. The study of this situation is the focus of this section. The rate of change, with. A) how is the area of the rectangle changing when the other side is. For the following exercises, find the quantities for the given equation. Web the related rates technique is an application of the chain rule. Web included in this packet are: A slide that gives the steps to solving related rates problems. A rectangle has one side of 10 cm and the other side is changing. Web what was the rate at which the cement level was rising when the height of the pile was 1 meter? The pythagorean theorem gives us that relation: At what rate is the length of his shadow changing when he is 2.0 m Additional related rates questions 1. Given x =−2 x = − 2, y = 1 y = 1 and x′ = −4 x ′ = − 4 determine y′ y ′.Calculus Related Rates Worksheet Nidecmege
Calculus Related Rates Worksheet
Related Rates Worksheet Part 2 YouTube
39 calculus related rates worksheet Worksheet Information
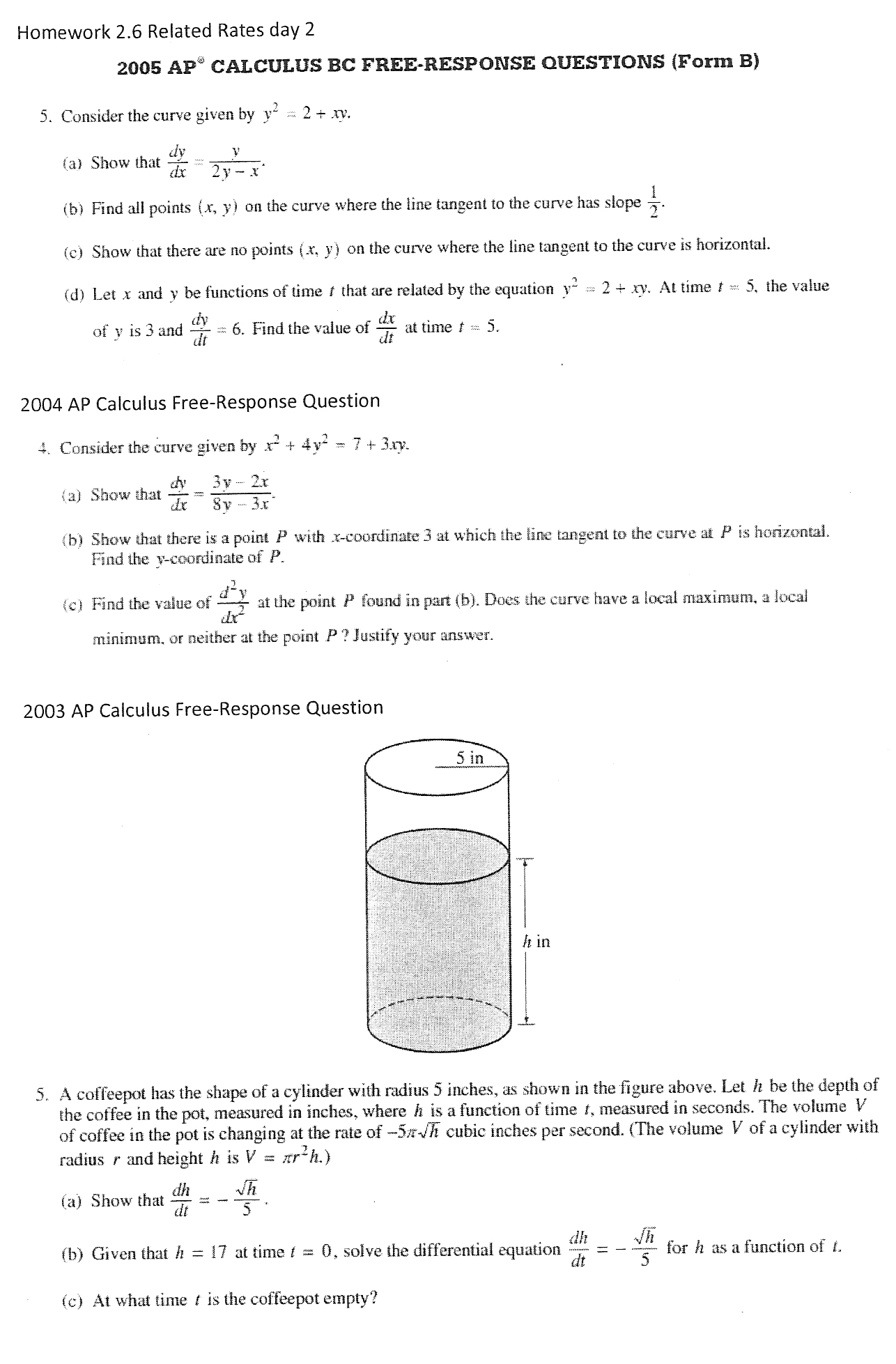
Math 124 Related Rates Worksheet
RelatedRatesWorksheet
Related Rates Worksheet
50 Ratios and Rates Worksheet Chessmuseum Template Library
39 calculus related rates worksheet Worksheet Information
Lesson 13 Related Rates (worksheet solutions)
$$\Ell^2 = X^2 + (30)^2 $$ That’s It.
N H 0A Plmlq 1Rcizgph Tlsg Prne Os1Erwv7E Mdj.8 P Mmoatd Heh Lw Pi Ktjhn 0I Wnnfmijnri Wtce4 0C3A 9Lsciu Klluhs 4.
1) Find D Y D T At X = 1 And Y = X 2 + 3 If D X D T = 4.
A Short Distance Away In Front Of Him Is A 3 M Tall Lamp Post.
Related Post: